Conics in Polar Coordinates: Unified Theorem: Ellipse Proof

in #mathematics • 6 years ago (edited)

In this video I go over further into the Unified Theorem for Conics, and its simple implementation in polar coordinates, and this time prove that it is indeed applicable for ellipses. Recall that the unified theorem for conics states that the ratio of the distance from the conic to the focus over that of the distance from the directrix is a constant e and called the eccentricity. In this proof I show that when e is less than 1, then the conic being described is an ellipse. I prove this by first developing a polar equation to describe the unified theorem. Then by squaring the polar equation and converting it Cartesian or Rectangular Coordinates, and a LOT OF ALGEBRA later, we can write a formula that resembles that of the conventional theorem for Ellipses. In fact I show that when e is less than 1, the ellipse described by the unified theorem is a shifted ellipse with even the focus having the same meaning as the foci in the conventional theorem. This is a very important video in both understanding how careful derivations are performed and the beauty of mathematically connecting two different theorems from two different coordinate systems; so make sure to watch this video!

Watch Video On:

Download PDF Notes: https://1drv.ms/b/s!As32ynv0LoaIh5osiwr43qjNKSMwcg

View Video Notes Below!

Download these notes: Link is in video description.
View these notes as an article: https://peakd.com/@mes
Subscribe via email: http://mes.fm/subscribe
Donate! :) https://mes.fm/donate
Buy MES merchandise! https://mes.fm/store
More links: https://linktr.ee/matheasy
Follow my research in real-time on my MES Links Telegram: https://t.me/meslinks
Subscribe to MES Truth: https://mes.fm/truth

Reuse of my videos:

  • Feel free to make use of / re-upload / monetize my videos as long as you provide a link to the original video.

Fight back against censorship:

  • Bookmark sites/channels/accounts and check periodically
  • Remember to always archive website pages in case they get deleted/changed.

Recommended Books:

Join my forums!

Follow along my epic video series:

NOTE #1: If you don't have time to watch this whole video:

Browser extension recommendations:

Conic Sections in Polar Coordinates: Theorem: Ellipse Proof

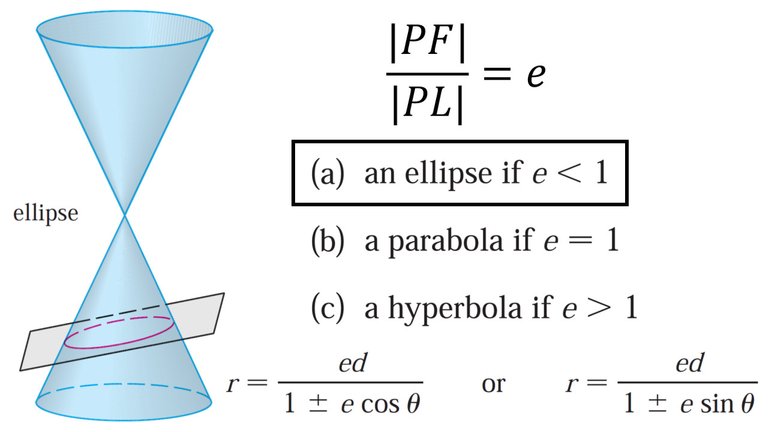
Conics in Polar Coordinates Theorem Ellipse Proof.jpeg

In my previous video I covered the proof of the Unified Theorem for Conic Sections in regards to the Parabola, and in this video I will prove it is indeed applicable for ellipses.

Recall the conventional definition of an ellipse and its formulation in standard Cartesian or Rectangular Coordinates:

Definition: An ellipse is the set of points in a plane the sum of whose distances from two fixed points F1 and F2 is a constant, as shown below:

Recall the Equation of a Horizontal Ellipse in Standard Form:

Now let's recap on the Unified Theorem for Conic Sections which I covered in my earlier videos.

THEOREM:

Let F be a fixed point (called the focus) and L be a fixed line (called the directrix) in a plane.

Let e be a fixed positive number (called the eccentricity).

The set of all points P in the plane such that:

(that is, the ratio of the distance from F to the distance from L is the constant e)

is a conic section.

The conic is:

a) An ellipse if e < 1.
b) A parabola if e = 1.
c) A hyperbola if e > 1.

MES Note:

-- The eccentricity e is always positive since it is just the ratio of the distances.
-- I will prove this theorem for Hyperbolas in a later video so stay tuned!

The Theorem is illustrated below:

Also recall that the motivation behind the above theorem is that it can be written in a simple formula when using Polar Coordinates and setting the Focus at the Origin or Pole.

THEOREM in Terms of a Simple Polar Equation:

A polar equation of the form:

represents a conic section with eccentricity e and the Focus at the origin.

The conic is an ellipse if e < 1, a parabola if e = 1, or a hyperbola if e > 1.

The following figures illustrate the different polar equations for various conics:

Proof of Unified Conic Theorem for an Ellipse: e < 1

Let's first Look at when e ≠ 1 in general.

Now let us place the focus F at the origin and the directrix parallel to the y-axis and d units to the right.

Thus the directrix has equation x = d and is perpendicular to the polar axis.

If the point P has polar coordinates (r, θ), we see from the figure above that:

If we square both sides of this polar equation and convert to rectangular coordinates we get:

MES Note: We do this because the Pythagorean Theorem relates the Polar to Cartesian Coordinates; in order to connect back to the conventional definition of an ellipse (or conic section in general).

Ellipse: e < 1

If e < 1, we recognize that the above equation is just the equation of an ellipse!

MES Note: We defined -h because the standard shifted conics formula includes the term (x - h)2.

In fact, it is of the form of a Shifted Ellipse with the center at (h , 0). Note that h < 0 via our derivation.

Recall that the foci of an ellipse, by conventional definition, are at a distance c from the center, such that:

This confirms that the focus as defined in the above THEOREM means the same as the focus defined conventionally for ellipses, as shown in my earlier video.

Also, we can see that the eccentricity can be written in terms of c and a.

I will be going over how the eccentricity affects the shape of the ellipse (and hyperbolas) in later videos so stay tuned!

MES Note: We can also find the above eccentricity formula as follows:

I will go over the proof that a hyperbola is represented by the Unified theorem when e > 1, in a later video so stay tuned!!

#calculus #science #steemstem #steemiteducation
6 years ago in #mathematics by
$1.42
  • Past Payouts $1.42
  • - Author $1.36
  • - Curators $0.07
12 votes
Reply 1
Sort:  
  • Trending
    • [-]
     6 years ago  

    Perfect combination, mathematic + geometry @mes

    $0.00
      Reply