Greetings to the entire academic and scientific community of steemit.
Introduction
We continue in the search of any type of movement that we can observe in our environment, the phenomenon of the movement we have demonstrated that it forms and it will form part of our existence and in equal form it is fundamental for the space-time that harasses us until the end of our lives, therefore, the interest of the humanity to know more and more on the different types of mobilities has not been in vain.
According to the above, we have become more and more linked to our nature or universe, getting to obtain knowledge unsuspected by humanity and in this way we have provided ourselves with greater stability and social benefits. Of course, when such learning has been implemented with coherence and wisdom, respecting the natural balance as much as possible, this characteristic constitutes the success or failure of any kind of discovery of any kind.
Until now we can mention that we have known mobilities such as; circular, parabolic, elliptical, hyperbolic, rectilinear-curvilinear, oscillatory, vibratory, undulating and chaotic, all of them with their particular characteristics and very useful for the development of our most daily activities or to obtain complex knowledge of our environment.
It is important to emphasize that each one of the previous mobilities has described a respective geometric place of a certain figure or geometric form, this last one generally gives the name to this movement, then, these geometric forms keep an intrinsic relation with the essential phenomenon of the movement, and in fact it has to be this way since our eyes reflect in our retina innumerable forms or figures with which our surroundings are constituted.
In this opportunity we will be analyzing the wonderful curve called cycloid, and the movement that it generates when any particle body or object passes through it, in a general way we can say that this curve is originated when a certain point which is on the edge of a circumference turns through a straight line without slipping in it, managing to configure the geometric place of this wonderful curve.
We must emphasize that this type of curve has fundamental properties among which we can mention its arc length, this represents eight (8) times the radius of that circumference that produces or originates it, another important property is related to the area under this curve, this is, the one enclosed between the arc that describes the cycloid and the abscissa axis, this area represents three (3) times the surface of the respective circumference that creates the cycloid.
But our purpose is to refer to the movement of a certain particle, body or object when transiting through the inverted cycloid curve, such mobility we will call cycloidal movement, and with this we must highlight two other properties of this figure such as brachistocrone and tautocrone.
When we refer to brachytochrone it is important to mention that this word is generated from the Greek word Braquisto, referring to the shortest and Chronos, at the time, the problem of brachytochrone was raised by the Swiss mathematician Johann Bernouilli, this statement would become one of the most popular challenges of our history.
The statement of this problem was focused in the following way; if we have two points, one A and another B, which are located in a certain vertical plane, taking into account that these points were not in the same vertical line and also were at different heights, therefore, for that time it was interesting to know the geometric place of this curve, through which a certain particle, body or (mobile) object would transit.
All the above expressed under the gravitational action and without presenting friction, where, starting from a state of rest or immobile from the mentioned point A, the mobile can reach the point B in the shortest time possible, originating in this way the phenomenon that we are interested in analyzing in this opportunity, that is, the cycloidal movement.
For the determination of this curve Johann Bernoulli implemented an analogy which was related to Fermat's principle, where he imagined a sphere falling by gravitational action through a non-homogeneous medium, therefore, the trajectory of this sphere would be refracted when passing from one medium to another.
In a general way, the curve we call cycloid was originated according to the above mentioned, and also giving origin to the approach, where we can express that not always the shortest way turned out to be the fastest one, this referring of course to the straight path, this characteristic is what I highlight the most of this extraordinary curve.
When referring to the property of the tautocrone, this word originates from the Greek Tauto, which means the same, and Cronus as we said is time, that is, the same time, this property is the most impressive of this curve, since when a ball or a certain mobile falls freely (in free fall) from any point of this curve, the time implemented during this fall is independent of the point from where the ball or mobile was released.
The previous action can be justified or determined by the fact that the slope of that curve is steeper at its ends, and therefore, when coming out from the top the mobile will be able to reach greater speed in a shorter time interval, that is, it will obtain greater acceleration, but when we start from any point further down the speed will be affected by the smaller space to travel and the capacity to accelerate will be less.
Cycloidal movement
The cycloidal movement is another phenomenon found in our environment and is characterized by the mobility of some particle, body or object (mobile) through the curve called inverted cycloid, it represents the geometric place that describes a certain point (P) which is located constantly (while describing this curve) at the edge of a radius circle (r).
This generating circle turns or spins on a straight line without slipping or sliding on it, this is done at a constant speed until this point (P) reaches the position whose coordinates are (2πR, 0), that is, in simpler words it is when this circle reaches to make a complete turn, of course, taking into account the point (P) as reference, then we will observe the trajectory of this curve in the following figure 1.

In the previous figure 1, we could observe the geometric place of the non-inverted cycloid described by the point (P), which is anchored to the generating circumference, but it is necessary to know the shape of an inverted cycloid as we will see in the following figure 2.
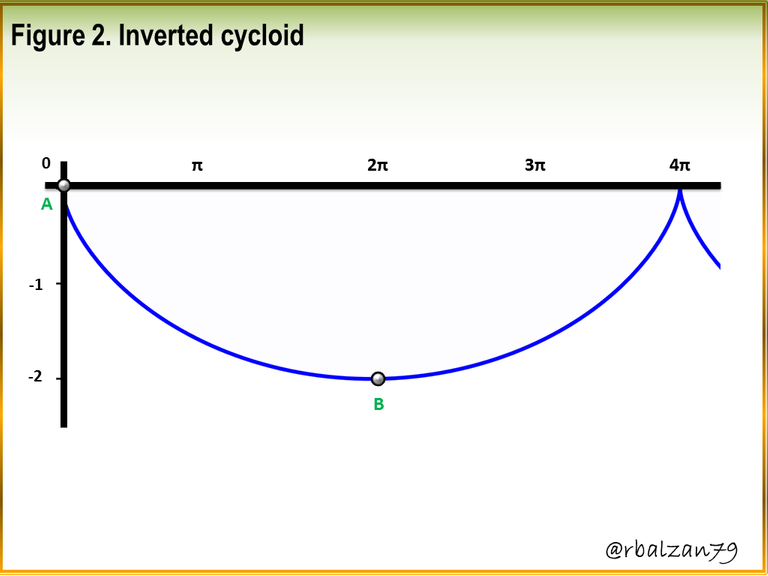
The previous figure 2, represents the type of cycloid implemented to carry out the cycloidal movement, it is important to take into account that for the analysis of the cycloidal movement developed by some particle, body or object, we must always take into account the important properties previously mentioned such as the Brachistocrone and the Tautocrone.
Before extending the properties already described, it is important to know how a non-inverted cycloid could be generated in our environment, and in this way we could verify that such mobility is part of any of our daily activities, An example of the generation of this non-inverted curve is shown in figure 3 below.
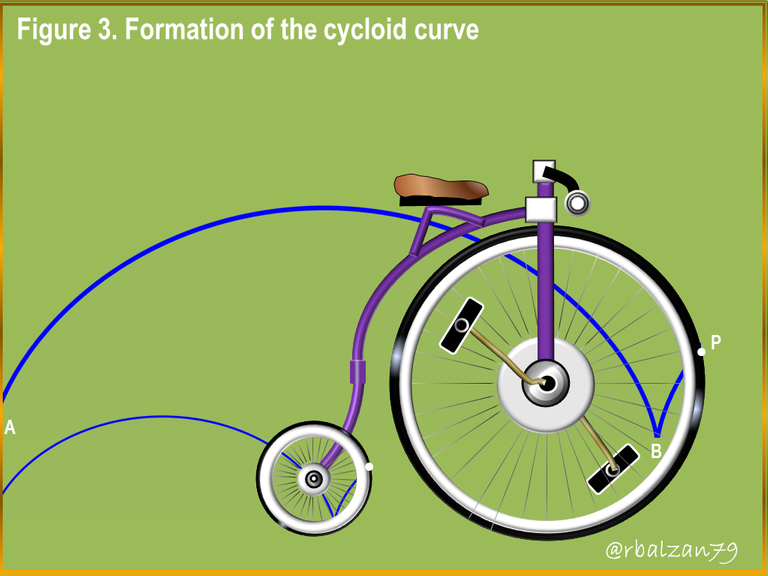
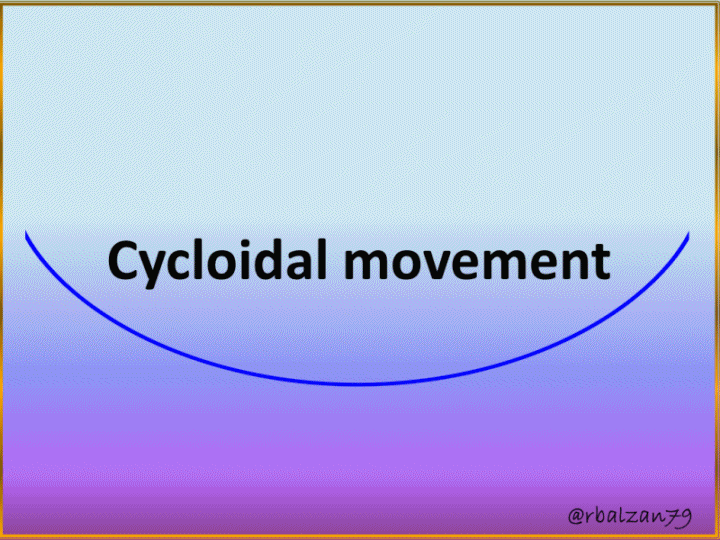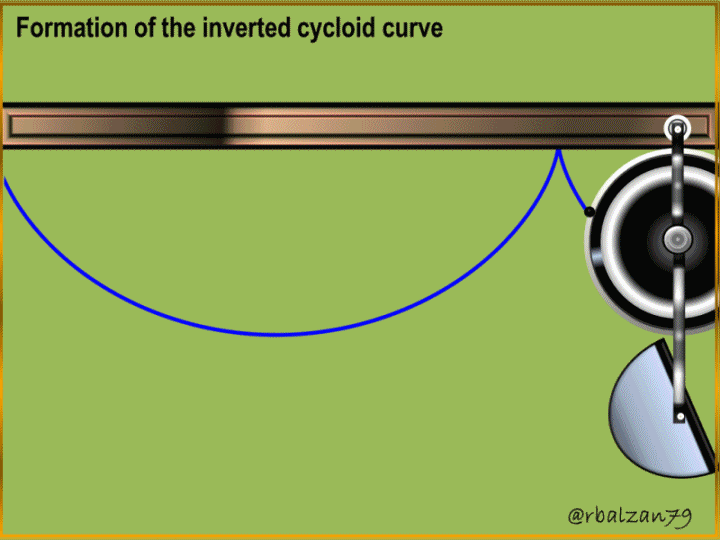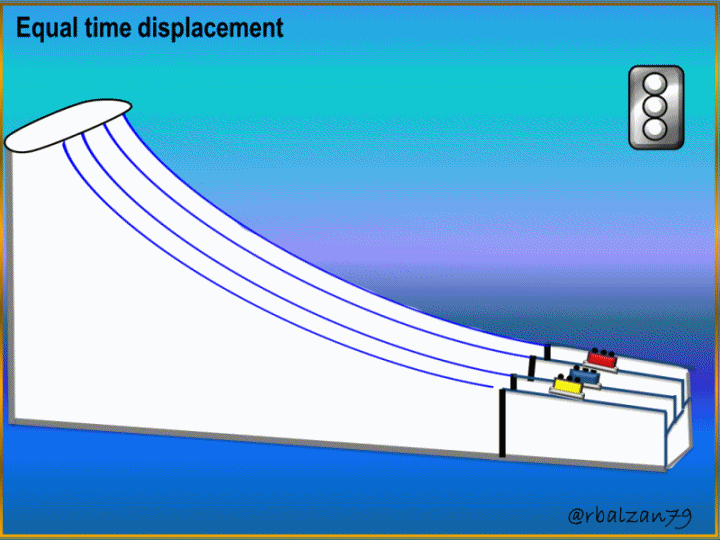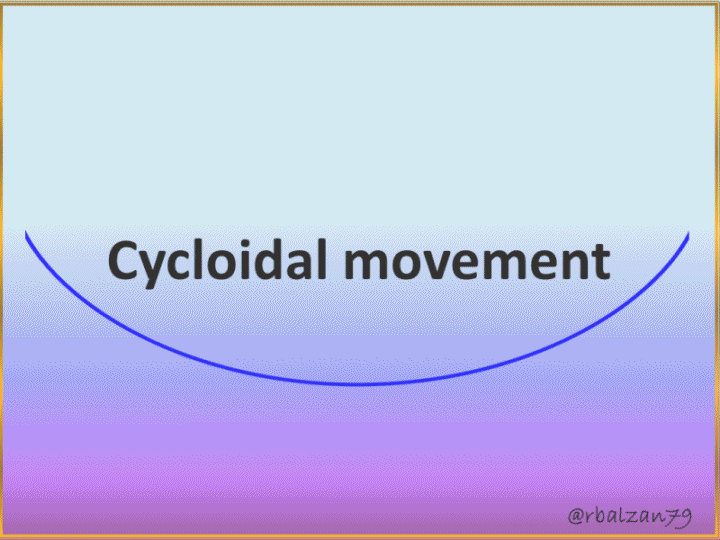
In this way we could find many examples of this type of non-inverted cycloid formation as observed in the previous figure 3, now it is also necessary to be able to visualize the formation or generation of an inverted cycloid through any mechanism present around us, therefore, next we will observe the generation of an inverted cycloid in the following figure 4.
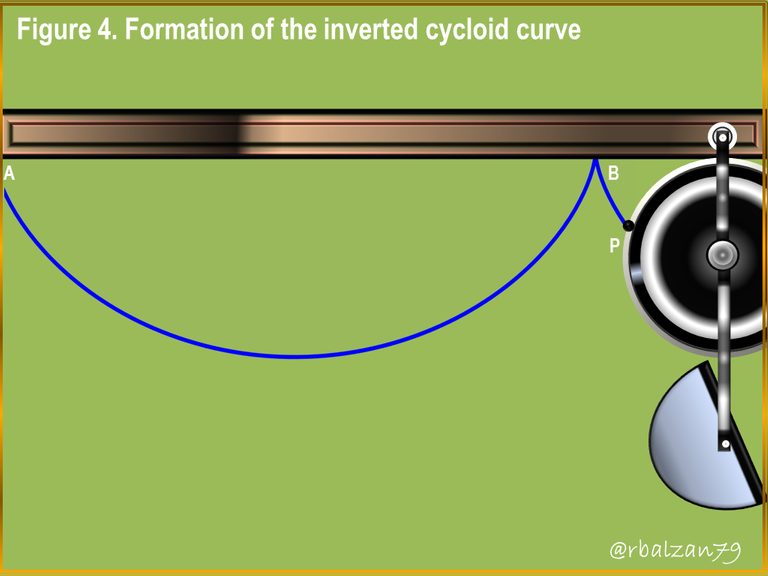
Like the previous example in figure 3, we could find ourselves in our environment with a great amount of activities that can generate or develop this type of inverted curve, as we could observe in the example in figure 4, and in this way continue to verify that the cycloidal movement is among us.
Now we can refer to the interesting properties of this inverted curve, starting with the Brachytochrone, for this we must remember Johann Bernouilli's statement, which focused on two points (A and B), which were located in the same plane but not in the same vertical line and at different heights, and according to the previous conditions should seek the curve to be transited by a given mobile from A to B implemented the shortest time possible.
That approach gave rise to the phenomenon that we know today as cycloidal movement, since the geometric location of this curve turned out to be that of the figure that we currently call the inverted cycloid, and therefore, the movement that takes place through it we call cycloidal, taking into account the origin of the word brachistocrone, where, Brachisto, refers to the shortest and Chrono in time, we will observe the following figure 5.
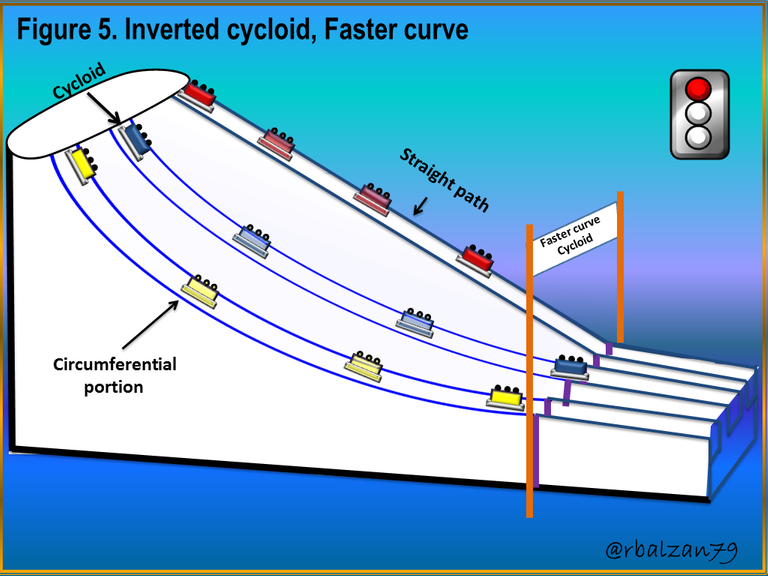
In the previous figure 5, we could observe that the trajectory of an inverted cycloid represents the shortest curve in transit from a point A to a point B, in relation to a portion of a circumference and a straight trajectory, all this with the initial conditions such as free fall, under the gravitational action and without friction, also proving that a shorter trajectory does not mean that it is the fastest.
When referring to the other property, that is, the tautochronous one, we can say that from the mobility point of view it is impressive the characteristics that this property gives to a certain mobile when it travels through an inverted cycloid, especially the implemented time, since when a certain mobile is freely dropped regardless of its departure point, the implemented time will be the same at the arrival point.
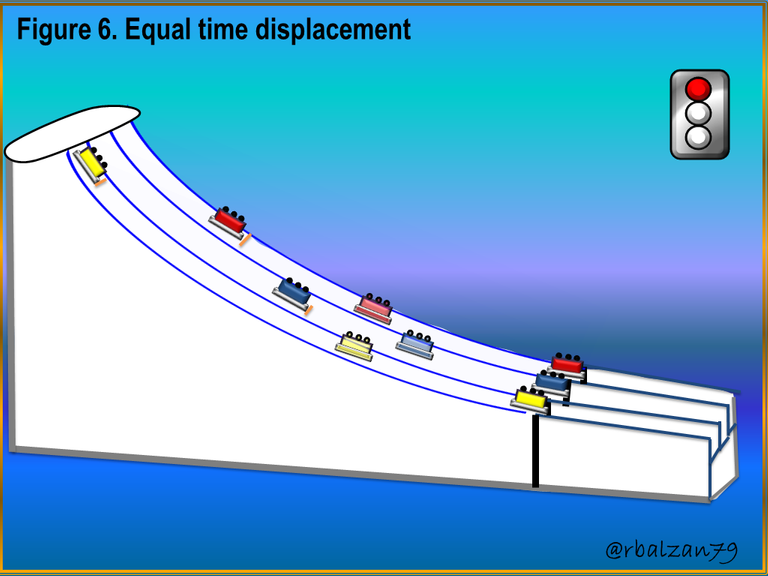
According to the aforementioned, regardless of the height from where any particle, body or object (mobile) is released or dropped, the time invested by said mobile will always be the same when reaching the end of the trajectory of the inverted cycloid as we can see in the following figure 6.
To close it is important to show the behavior between a cycloidal pendulum and a simple pendulum, where, the internal structure that frames these pendulums was designed with the implementation of three cycloid curves as we can see in the following figure 7.
Figure 7. Behavior between a cycloidal pendulum and a simple one
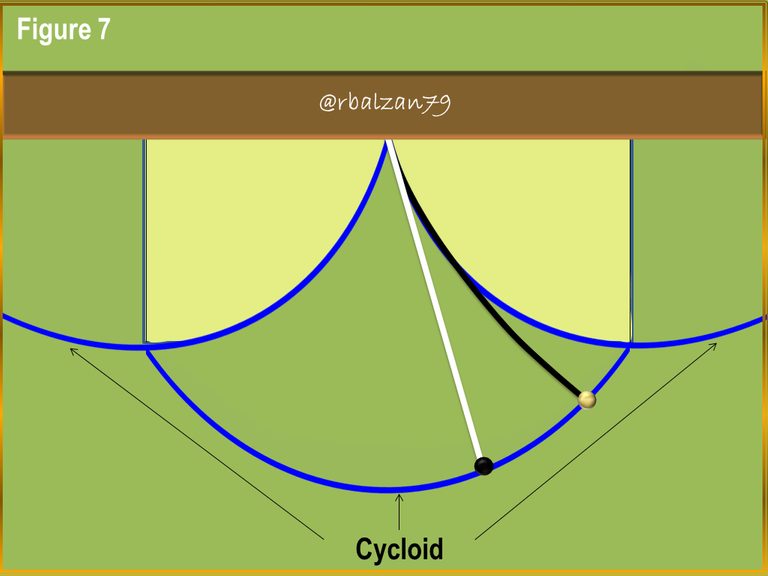
From figure 7 we can express that even though the cycloidal pendulum makes a greater travel it passes through the equilibrium point at the same time as the simple pendulum as can be seen in the gif at the beginning of this article, showing how wonderful this cycloidal curve is.
Conclusion
We continue to see that the phenomenon of movement is among us at any moment in our lives, and without it we could say that not even the universe itself would exist. This essential characteristic is what has exponentially increased man's interest in knowing more and more about this phenomenon, and we can observe this throughout our history.
In our case we continue with the identification of any type of mobility that we can observe in our daily environment, remember that we have already analyzed important and fundamental mobilities such as; the circular, parabolic, elliptical and hyperbolic movement, these movements are essential for the understanding of any other type of this phenomenon, like those described in previous articles like the oscillatory, vibratory, among others.
In this opportunity we could know in a general way the cycloidal movement and the curve that generates such mobility as the cycloid above all the inverted one, where this cycloid curve, represents the geometric space generated by a certain point (P) of a circumference that turns or spins on a straight line without slipping or sliding at constant speed, as we could observe especially in the figures 1 and 3.
From the inverted cycloid, we could know that it represents the fastest geometric place or space to transit from a certain starting point (A) to a finishing point (B), which could be found in the same plane but not in the same vertical line and at different heights, taking into account initial conditions such as; free fall (without thrust), under the effect of gravitational action and without any kind of friction during the whole journey.
But we could also observe the Tautochronous property of this inverted figure, which allows an equal period of time for a mobile that starts the route from the highest end of this curve as for the one that starts the route lower, even when releasing two mobiles in the opposite direction, one higher than the other, both will be exactly in the center of the curve at the same time.
In short, when we release three mobiles in free fall following different trajectories, the inverted cycloid curve will be covered in less time (Figure 5). If we release these three mobiles at different initial heights, each one following an inverted cycloid, they will take the same time to reach the end point of the curve (Figure 6).
Until another opportunity my appreciated friends and readers of steemit, very specially to the members of the big communities friends of #steemstem and #curie, reason why I recommend widely to be part of these exemplary projects, because they emphasize the valuable work of the academy and the scientific field.
Note: All images were made using the Power Point application and the animated gif with the PhotoScape application.
Bibliographic References
[1]Charles H. Lehmann. Geometría analítica.[2]THE CYCLOID
Congratulations @rbalzan79! You have completed the following achievement on the Steem blockchain and have been rewarded with new badge(s) :
You can view your badges on your Steem Board and compare to others on the Steem Ranking
If you no longer want to receive notifications, reply to this comment with the word
STOPTo support your work, I also upvoted your post!
Do not miss the last post from @steemitboard:
@tipu curate
Upvoted 👌 (Mana: 10/15 - need recharge?)
Thanks for the support, @ritch. Greetings.
Excellent approach to the Cycloidal movement, thanks for sharing with all the STEEM community @rbalzan79
Thank you for your comment @carlos84. Greetings.
This post has been voted on by the SteemSTEM curation team and voting trail. It is elligible for support from @curie and @minnowbooster.
If you appreciate the work we are doing, then consider supporting our witness @stem.witness. Additional witness support to the curie witness would be appreciated as well.
For additional information please join us on the SteemSTEM discord and to get to know the rest of the community!
Thanks for having used the steemstem.io app and included @steemstem in the list of beneficiaries of this post. This granted you a stronger support from SteemSTEM.
Thanks to the communities of @SteemSTEM, @curie y @minnowbooster for the support received.