A Mathematical Genius Amidst the Adversities of his Time
It was on 6 April 1829 that the great Norwegian mathematician Niels Henrik Abel died in the Norwegian city of Froland, as a result of tuberculosis, one of the most lethal diseases of the time. Despite his short life, Niels Henrik Abel made an enormous amount of mathematical contributions that proved essential in producing profound advances in fundamental areas of mathematics such as algebra and mathematical analysis. It is unfortunate that Abel, like many great mathematicians in history, received recognition after his death, where his mathematical genius is now recognised in many scientific concepts, as well as in many outstanding scientific awards.
This great exponent of Norwegian mathematics is mainly known in the mathematical and scientific world for his work proving the irresolvability of the quintic equation of the fifth degree by rationals, but Abel also made extensive contributions that proved to be pioneering in the study of elliptic functions and even functions that have been called ‘Abelian functions’, in his honour.
Main Mathematical Contributions.
- The Mathematical Proof of the Impossibility of Solving the General Quintic Equation by Radicals:
Niels Abel succeeded in constructing a mathematical proof that it is not possible to find a general formula involving only arithmetic operations and radical operations that is capable of expressing the solutions of a polynomial equation of the fifth degree (or higher) in terms of its coefficients. This momentous result served to close a mathematical problem that had remained open for centuries.
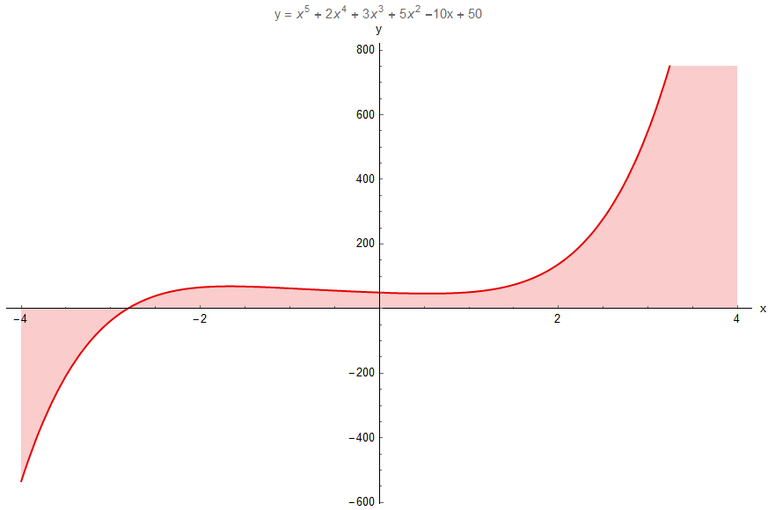
Plot of the 5th-Degree Equation: x5 + 2x4 + 3x3 + 5x2 - 10x + 50.
Abelian Group Theory:
It is necessary to clarify that the term ‘Abelian groups’ was coined later in his honour, but it is also undeniable how fundamental his works were in the detailed study of these commutative groups. Moreover, all his mathematical explorations in the study of the solvability of different types of polynomial equations were intrinsically related to algebraic structures, more specifically to group structures.Construction of Elliptic and Abelian Functions:
Abel carried out a foundational work related to the study of elliptic functions, which stands out for the discovery of impressive mathematical properties pertaining to this type of functions, and all this independently of another great exponent in the development of the theory of elliptic functions such as the great German mathematician Carl Gustav Jacob Jacobi.Investigations in Power Series:
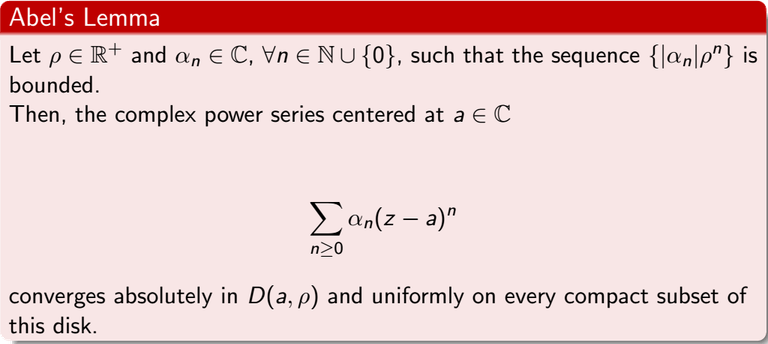
As for his contributions to mathematical analysis, Abel's mathematical genius achieved remarkable results in the study of the convergence of power series, and all this under mathematical rigor of the highest level. For example, Abel's theorem, which is intrinsically related to 'the convergence of series at the edges of their convergence interval', turns out to be a fundamental result for the analysis in |R.
Life and Performance.
Born in the Norwegian city of Nedstrand on August 5, 1802, Niels Henrik Abel was a young man who, despite suffering serious economic difficulties within his family, this did not limit him from being able to show his exceptional talent for mathematics from a very early age. Abel carried out his undergraduate studies at the Royal Frederick University (now the University of Oslo) where his professors would quickly notice his genius, which is why he would manage to obtain a scholarship that would take him to travel through Europe, where he would meet some of the most relevant mathematicians of the time.
But economic adversities always had a high presence in his life, since despite achieving important mathematical discoveries that he would carry out, Abel always had to fight to find a permanent position in academia. reason that led him to face a stoning poverty.
Key Publications:
'Mémoire sur les équations algébriques où l'on démontre l'impossibilité de la résolution de l'équation générale du cinquième degré' (Memoir on the Algebraic Equations Demonstrating the Impossibility of the Solution of the General Equation of the Fifth Degree) (1824):
Although initially this work would go unnoticed by the most relevant mathematicians within the European community of the time, in this work was his famous mathematical demonstration of the impossibility of finding a general solution by radials for the general quintic equation. and this is partly motivated by his poverty, which would lead him to not be able to publish his results adequately.'Recherches sur les fonctions elliptiques' (Research on Elliptic Functions) (Posthumously published in the year 1830):
This work would be fundamental to lay the foundations of the theory of elliptic functions.Numerous Articles published in the Scientific Journal 'Crelle's Journal':
Multiple publications were made in Crelle's Journal, which was an important mathematical journal of the time, where a large part of his research in algebra and analysis in R was covered.
Although poverty and lack of resources was common in his life, this did not prevent Niels Henril Abel from deploying all his mathematical genius, and achieving recognition, which, although posthumous, today is recognized as one of the most important mathematicians in history. Abel's life invites us to reflect on how important economic and financial stability is in a person's life, regardless of the level of talent to carry out a certain occupation, without a doubt economic stability and access to material resources are essential for the correct development of any career or occupation that any person wants to carry out.
One reason why, to cite one example, the members of the Paris Academy of Sciences ignored his works, something that frustrated him greatly, was that due to Abel's scarcity of resources, he was unable to adequately publish his mathematical findings. In addition to the lack of resources, he also did not have enough institutional support to help him have greater access to the most influential academic circles within mathematics of his time.
Unfortunately, Abel's premature death prevented him from continuing his crusade to spread his ideas and achieve a greater dissemination of his work, which to this day remains a cornerstone of modern mathematics.
For all of the above, thank you Abel.

Some Abel's Contributions.


Note: All the images related to Abel's Contributions are crafted by me using the text editor based on LaTeX: Beamer. Also, the plot about the quintic general equation shown in this post was created by me using the Wolfram language: 'Mathematica'.
'The Structure of this article is by my authorship too.'
Regards.
References
Weisstein, Eric W. "Abel's Convergence Theorem." From MathWorld--A Wolfram Web Resource. https://mathworld.wolfram.com/AbelsConvergenceTheorem.html
Fraleigh, J. B. A First Course in Abstract Algebra, 7th ed. Reading, MA: Addison-Wesley, 2002.
Weisstein, Eric W. "Abel's Lemma." From MathWorld--A Wolfram Web Resource. https://mathworld.wolfram.com/AbelsLemma.html
