Note: This text is not a part of the series about physics intended to be simple. It shows all the calculations needed to get the result. If you get scared by math, this post isn't for you ;)
Note 2: Since there is no LaTeX on Steemit, I resorted to Unicode for representing the equations. If they aren't readable enough - my apologies.
A few months ago, while browsing the internet, I stumbled upon a thread which looked like a typical question asked by someone interested in science, and turned out to be a really interesting problem.
The question that has been asked concerned the shape of a black hole. A few people replied that the event horizon (the boundary of a black hole, from beyond which there is no escape) has the shape of a ball (which should be actually described as a sphere, since the horizon is a 2-dimensional surface, and not a 3-dimensional shape). Someone suggested that it's not exactly true, because black holes usually spin, which flattens them. I entered the thread then and said that even when a black hole is spinning, its horizon is still spherical - it's described by an equation like r = const. But is that really so...?
When I wrote my response, I had a hunch. In the case of a Schwarzschild black hole, r = const actually describes a sphere, as it is a spherically symmetric solution. In the case of a rotating black hole (called a Kerr black hole, from the physicist who first derived the metric describing them) it doesn't have to be so. r is just one of the coordinates, which can be assigned to the space arbitrarily and the points with the same r don't have to lie on a sphere. In order to be sure, we have to calculate it.
One of the ways of describing a surface is a so-called metric - it describes a way of calculating distances between points with given coordinates (I wrote a bit more about it in my series of articles about the mathematics of relativity). It's a fundamental tool in the General Theory of Relativity - the properties of space-time are described there exactly with a metric. Spinning black holes are described, as I already mentioned, by the Kerr metric:
g = (1 - 2Mr/Σ) dt² - Σ/Δ dr² - Σ dϑ² - (r² + a² + 2Mra² sin² ϑ / Σ) sin²ϑ dφ² + 4Mra sin² ϑ / Σ dtdφ
where:
M- the black hole's massa- the black hole's angular momentum (divided by mass - there is a limita ≤ M)t,r,ϑ,φ- space-time coordinatesΔ = r² - 2Mr + a²Σ = r² + a² cos² ϑ
(everything is in units of c = G = 1, for simplicity; if we put GM/c² instead of M and ct instead of t, we would get SI units)
The event horizon (actually, two of them!) appears in the place where Δ = 0 - so r = M ± √(M² - a²). We are only interested in the outer horizon, as it is what decides the shape of the black hole.
Since we can give a concrete value for r, which corresponds to the horizon, it means that the horizon is described by r = const. We aren't interested in the evolution in time, either (and there is none - the Kerr metric is stationary), so we also assume t = const. This means that in order to limit the metric to the horizon itself, we only have to set dr = dt = 0. Such a metric (after additionally changing its sign - the spatial part of the metric is negative, but it doesn't matter when we don't mix space with time, and we are only concerned with space here) would look like this:
h = Σ dϑ² + (r² + a² + 2Mra² sin² ϑ / Σ) sin²ϑ dφ²
It's not very beautiful, but much more pleasant than the full metric.
Now we use the fact that we know the precise value of r: r = M + √(M² - a²). We then get:
r² + a² = 2Mr(becauseΔ = 0)= 2M (M + √(M² - a²))Σ = r² + a² cos² ϑ = r² + a² - a² sin² ϑ = 2M (M + √(M² - a²)) - a² sin² ϑ
The metric can then be written as:
h = 2M² [ (1 + √((1 - a²)/M²) - a²/(2M²) sin² ϑ) dϑ² + (1 + √((1 - a²)/M²))²/(1 + √((1 - a²)/M²) - a²/(2M²) sin² ϑ) sin² ϑ dφ²]
Well, that's worse, but we can simplify it a bit. First, we can drop the leading 2M². It only controls the size of the horizon, but not its shape - so we don't care about it, and if we need it, we can always restore it. Second, we have a/M in many places - let's denote this by α. As I already mentioned, there is a limit of a ≤ M, which means that 0 ≤ α ≤ 1 (0 will get us back to the Schwarzschild metric, 1 is so-called extremal Kerr). This brings the metric to the following form:
h' = (1 + √(1 - α²) - 1/2 α² sin² ϑ) dϑ² + (1 + √(1 - α²))²/(1 + √(1 - α²) - 1/2 α² sin² ϑ) sin² ϑ dφ²
Let us also denote 1 + √(1 - α²) by β, because why not, and divide the whole metric by β (again - just a matter of scale):
h'' = (β - 1/2 α² sin²ϑ)/β dϑ² + β/(β - 1/2 α² sin² ϑ) sin² ϑ dφ²
And, finally: we denote the ugly fraction (β - 1/2 α² sin²ϑ)/β by ξ(ϑ):
h'' = ξ(ϑ) dϑ² + 1/ξ(ϑ) sin² ϑ dφ²
When ξ(ϑ) is identically equal to 1 (and it is when α = 0, so in the Schwarzschild case), this metric describes a sphere. Everything is fine up to now :)
What do we do, when ξ is not 1, though? It is tempting to just call ϑ and φ something like latitude and longitude, but it would be the same mistake as saying that constant r is a sphere - we have no guarantee that those coordinates have some physical interpretation. What's left is to generate a surface with an equivalent metric.
We will describe our generated surface by a parametrization: x(ϑ, φ), y(ϑ, φ), z(ϑ, φ). Such a form means that every point on the surface is described by a pair of coordinates (ϑ, φ). For every such pair we can then calculate corresponding 3D coordinates. For example, we could parametrize a sphere like so:
x(ϑ, φ) = r sinϑ cosφ
y(ϑ, φ) = r sinϑ sinφ
z(ϑ, φ) = r cosϑ
Since we still have a rotational symmetry in the Kerr metric, fortunately, we can use a trick: we say that z is the axis of symmetry, and we transform x and y into the distance from the z axis (denoted by ρ) and an angle φ - so we basically introduce cyllindrical coordinates. This lets us write:
x(ϑ, φ) = ρ(ϑ) cosφ
y(ϑ, φ) = ρ(ϑ) sinφ
z(ϑ, φ) = z(ϑ)
(In the case of a sphere, we would have ρ(ϑ) = r sinϑ, z(ϑ) = r cosϑ).
Now we need to calculate the metric of our surface. The metric of a flat 3D space in the x, y, z coordinates is very simple: dx² + dy² + dz². We just have to limit it to our surface and express it using ϑ and φ.
We will do it by transforming the differential forms to the new coordinates:
dx = ∂x/∂ϑ dϑ + ∂x/∂φ dφ
dy = ∂y/∂ϑ dϑ + ∂y/∂φ dφ
dz = ∂z/∂ϑ dϑ + ∂z/∂φ dφ
Let's calculate:
dx = dρ/dϑ cosφ dϑ - ρ(ϑ) sinφ dφ
dy = dρ/dϑ sinφ dϑ + ρ(ϑ) cosφ dφ
dz = dz/dϑ dϑ
After substituting this in the original 3D metric:
dx² + dy² + dz² = [ (dρ/dϑ)² + (dz/dϑ)² ] dϑ² + ρ(ϑ)² dφ²
We would like this to be equal to the h'' metric. For it to be like this, the following must hold:
ρ(ϑ)² = 1/ξ(ϑ) sin² ϑ
(dρ/dϑ)² + (dz/dϑ)² = ξ(ϑ)
The first condition gives us: ρ(ϑ) = sinϑ/√ξ(ϑ). We can calculate the derivative, which gives:
(dρ/dϑ)² = cos² ϑ / ξ(ϑ)³
(dz/dϑ)² = ξ(ϑ)(1 - cos² ϑ)/ξ(ϑ)⁴
The second equation is an ordinary differential equation for z(ϑ) - it can be solved numerically, and we will have the full description of our surface.
So now - what does such a surface look like? Let's note that when we calculate (ρ(ϑ), z(ϑ)) for various values of ϑ between 0 and π, we get x and z coordinates of points on our surface for φ = 0 - so it's something like a "zero meridian". We just rotate the resulting curve around the z axis and voila! We have our surface.
Let's try it. Shown below are the "zero meridians" for α = 0 (which should be half a circle - the Schwarzschild case, a sphere), α = 0.5 and α = 0.8:
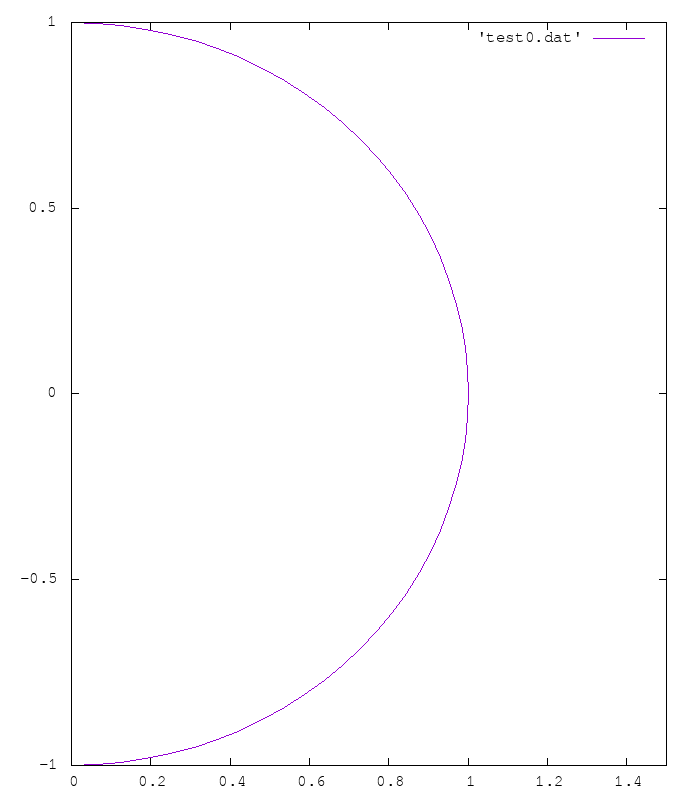
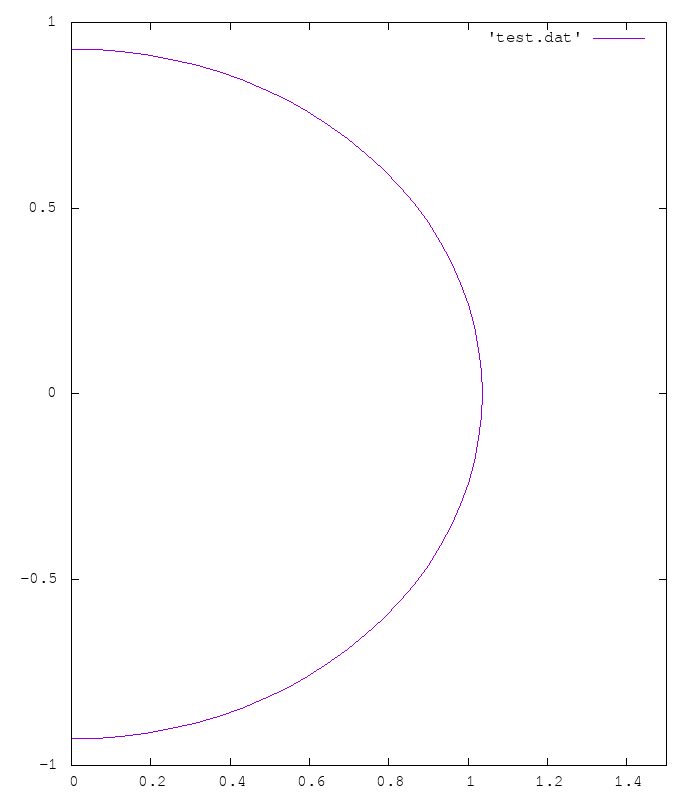

If you look closely, you can see some flattenning for α=0.5, and quite a bit of it for 0.8.
And what happens for larger values of α...? I tried to see - and the program calculating z(ϑ) numerically started throwing complex numbers at me. What the...?
I started suspecting a mistake in the calculations, so I checked them multiple times, but everything looked OK. I concluded, then, that maybe this is the correct result - but what would be its interpretation?
The problem stems from the way of calculating dz/dϑ - namely, we take a coefficient of the surface's metric that multiplies dϑ², and we subtract (dρ/dϑ)² from it. Well, but for large enough values of α, the second part turns out to be larger than the first one, and the square of the derivative of z turns out negative.
But wait... what is larger than what here? The first part is a coefficient of the metric, which is basically a square of the length of the line that we draw by moving along the surface by dϑ. The second one is the square of the change in our distance from the axis of rotation when we move like that. A negative result means that by moving along the surface a bit, we move away from the axis of symmetry by more than we move along the surface - WTF? Such things shouldn't happen, should they?
Well, it turns out that, although counter-intuitive, it's actually possible. The source of the problem is that we try to divide the horizon into circles - circles of latitude (constant ϑ), and we try to "stack" them along the z axis so that the distances on the surface created that way were the same as on the horizon. However, if the geometry of space is sufficiently curved, the curvature of circles of latitude can vary in a way completely independent from the distance on the surface - and this is what happens here. Our distance from the axis of rotation (ρ) is just the radius of curvature of the circle of latitude, and it varies so fast that the distance on the surface "doesn't catch up". Such a situation is possible in a curved space, but not in a flat one - like the one we live in and try to picture the shape of the horizon.
So, simply put: the shape of the horizon is something that just doesn't exist in a flat space. Oh well. We won't see what an extremal horizon looks like (α = 1; my master's thesis was actually about such horizons).
We won't be able to see the actual shape of the horizon - but an observer near such a black hole would surely see something, right? He could take a picture and print it on a 2-dimensional piece of paper - what would be the result?
A black hole strongly deflects rays of light that pass near it, and absorbs some of them - as a result, an observer sees a black spot on the sky, surrounded by a distorted image of the background. The shape of this spot - called a "shadow" of the black hole - is not the shape of the horizon, though. It is determined by how the black hole affects light. When the black hole is spinning, light passing it on the one side is deflected a bit differently, than on the other side, which causes the image to be a bit flattened, indeed - but only on one side, and in the direction perpendicular to the axis of rotation, not parallel.

The picture to the right is a result of simulating a black hole with α ≈ 0.95. The axis of rotation is vertical here. You can clearly see the flattened left side - caused by the fact that light on this side can pass a bit closer to the black hole without falling in, than on the other side.
In summary: I haven't expected that some horizons can't be pictured in 3D - which is kind of a consequence of my being sure that the horizon is always a sphere ;) A random person on the internet asked a simple question, and I actually learned a lot from it. This was a fascinating experience :)
References
I've actually discovered this one after I've done all the calculations, but not surprisingly, it is actually a well-known result that the surface of the horizon cannot be embedded in a flat 3D space for α > √3/2:
L. Smarr Surface Geometry of Charged Rotating Black Holes, Phys. Rev. D 7, 289 (1973)
Congratulations @ebvalaim! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOPCongratulations @ebvalaim! You have received a personal award!
Click on the badge to view your Board of Honor.
Do not miss the last announcement from @steemitboard!